
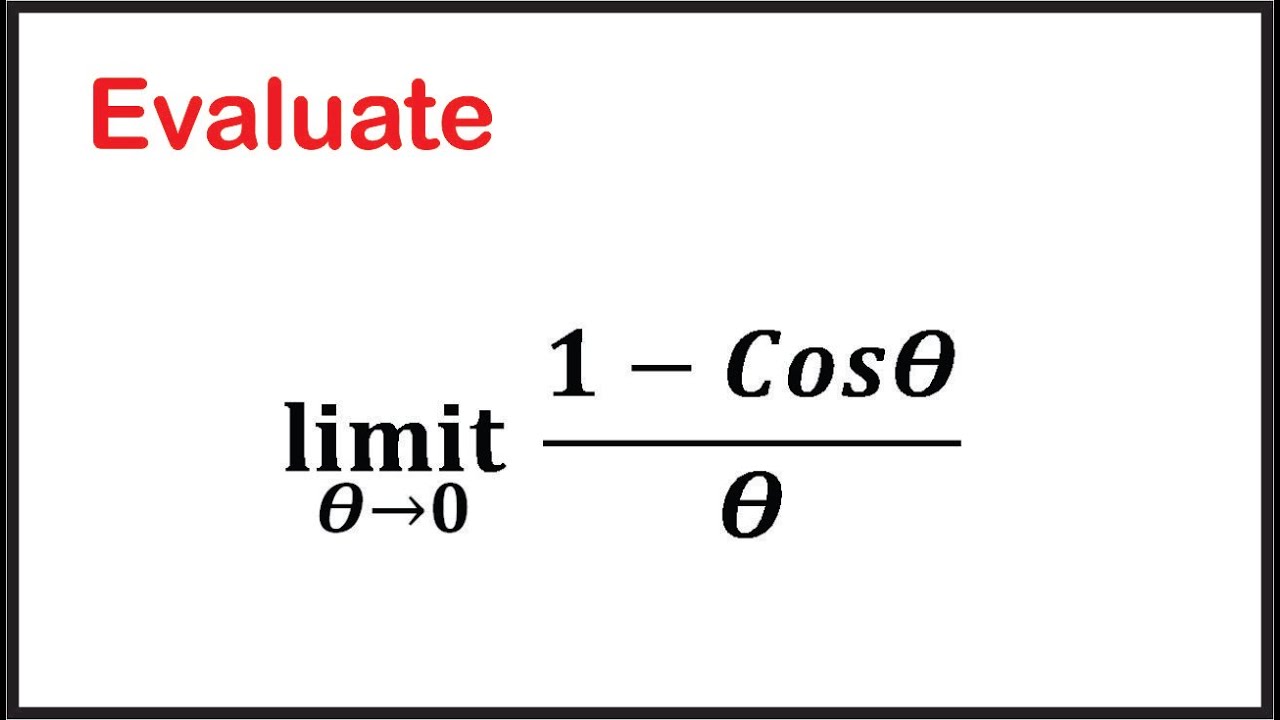
Using trigonometric identities is another clever way to manipulate functions so that we can more easily evaluate the limit. If x is allowed to decrease without bound, f(x) take values within and has no limit again.Lim x → c f ( x ) = L \lim_ = 1 lim x → 0 x s i n ( x ) = 1. The sine function has a range from displaystyle 0,1, which means that the range must be. For example, take the function f (x) 2x f (x) 2x, graphed below. For, if a sequence of values of the variable x approaches c as a limit (Definition 2.1), then a sequence of values of the function f(x) x will also approach c. Given a function f f, a limit is the value that f (x) f (x) approaches as x x approaches some value.
LIMITS IN CALCULUS HOW TO
If x is allowed to increase without bound, f(x) take values within and has no limit. Recall the Squeeze theorem can be used to solve for the limit. Understanding how to do limits in calculus is crucial for understanding other fundamental concepts in calculus, such as differentiation and integration.
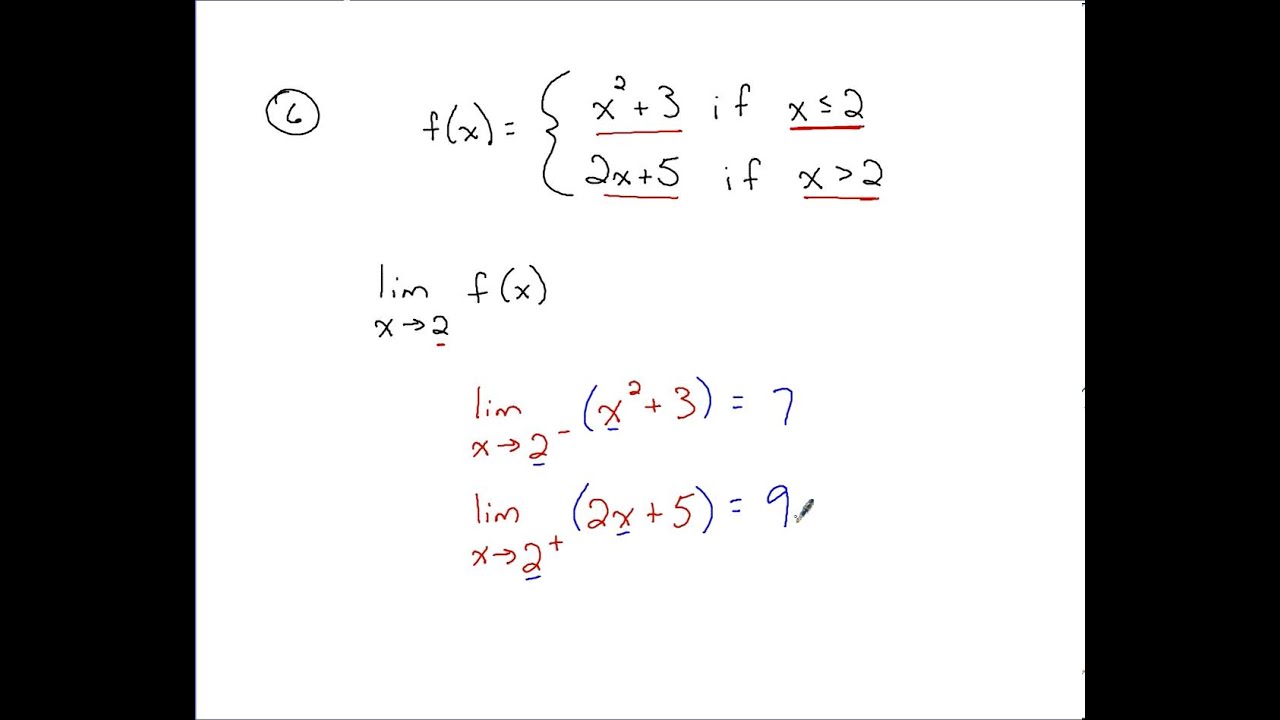
The graph below shows a periodic function whose range is given by the interval. These are symbols used to indicate that the limit does not exist. The limit applies to where the lines on the graph fall, so as the value of x changes, the number value will be where the limit (line) and x value intersect. One common graph limit equation is lim f(x) number value.

This is read as: the limit of fx() as x approaches a. The definition of sequence itself is vague The usual examples are not enough to develop intuition I hope this question is not too vague. In graphs, calculus works with this simple definition of limits and applies it to equations. Our study of calculus begins with an understanding of the expression lim x a fx(), where a is a real number (in short, a ) and f is a function. Most of the time, math limit formulas are the representation of the behaviour of the function at a specific point. It is one of the basic prerequisites to understand other concepts in Calculus such as continuity, differentiation, integration limit formula, etc. Note that - ∞ and + ∞ are symbols and not numbers. Limits and Continuity 2.1: An Introduction to Limits 2.2: Properties of Limits. Limits math is very important in calculus.

To understand what limits are, lets look at an example. This simple yet powerful idea is the basis of all of calculus. We writeĪs x approaches - 2 from the right, f(x) gets larger and larger without bound and there is no limit. Limits describe how a function behaves near a point, instead of at that point. This graph shows that as x approaches - 2 from the left, f(x) gets smaller and smaller without bound and there is no limit. In this example, the limit when x approaches 0 is equal to f(0) = 1. Note that the left and right hand limits are equal and we cvan write Note that the left hand limit and f(1) = 2 are equal. Note that the left and right hand limits and f(1) = 3 are all different.
The graph below shows that as x approaches 1 from the left, y = f(x) approaches 2 and this can be written asĪs x approaches 1 from the right, y = f(x) approaches 4 and this can be written as We consider values of x approaching 0 from the left (x 0). In fact we may talk about the limit of f(x) as x approaches a even when f(a) is undefined.Įxample 2: Let g(x) = sin x / x and compute g(x) as x takes values closer to 0. NOTE: We are talking about the values that f(x) takes when x gets closer to 1 and not f(1). In both cases as x approaches 1, f(x) approaches 4. We first consider values of x approaching 1 from the left (x 1). Example 1: Let f(x) = 2 x + 2 and compute f(x) as x takes values closer to 1.


 0 kommentar(er)
0 kommentar(er)
